Additional comments
Forces and Acceleration
As mentioned in the video, we talk about forces and accelerations interchangeably. The arrows in the video always show acceleration – the longer the arrow, the larger the acceleration. The force can be deduced by the formula F=ma. So for example the middle part of the Earth model, which is assumed to be more massive, requires larger forces for the same acceleration.
Interestingly, fictitious forces all have a property that they are proportional to the object’s mass. That is the more massive an object, the larger the fictitious force that will be applied on it. Therefore, the acceleration due to a fictitious force doesn’t depend on the object’s mass.
The same is true for gravity. The acceleration due to gravity is independent of the mass. This is one of the reasons that makes Gravity suspicious as a fictitious force also, as, to a certain extent, it is perceived in General Relativity.
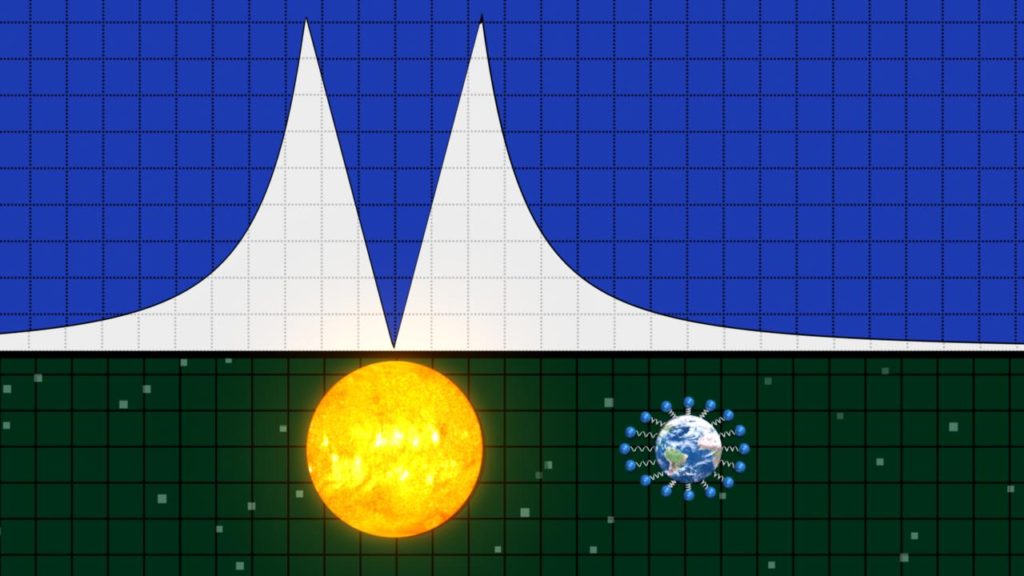
Gravity's graph
In the video the gravity graph stops at the Sun, but actually we can continue it inside the Sun as well.
This is how it would look like if the Sun had uniform density, according to Shell’s theorem:
Is that really how tides are generated?
The video shows accurate simulations but with very simple bodies. It accurately computes the tidal forces that are generated, and how the simulated bodies react to them.
The beads in the video simply stretch according to the direction and magnitude of the tidal forces that act on them. So they are useful in visualizing this effect.
However, different types of bodies react to tidal forces differently. It usually claimed the oceans are mostly driven by the tangential part of the tidal forces (i.e., those parts of the tidal forces tangent to the Earth surface), and that this is the main cause of tides on Earth.
The Earth’s rock itself also responds to tidal forces, but it is actually more driven by the radial part of the tidal forces (tidal forces pulling towards or away from the Earth’s center).
Also, the tide phenomenon itself is very much affected by many other factors, such as the shapes of the shorelines and other factors.
